Thieves and detectives

Epsilons

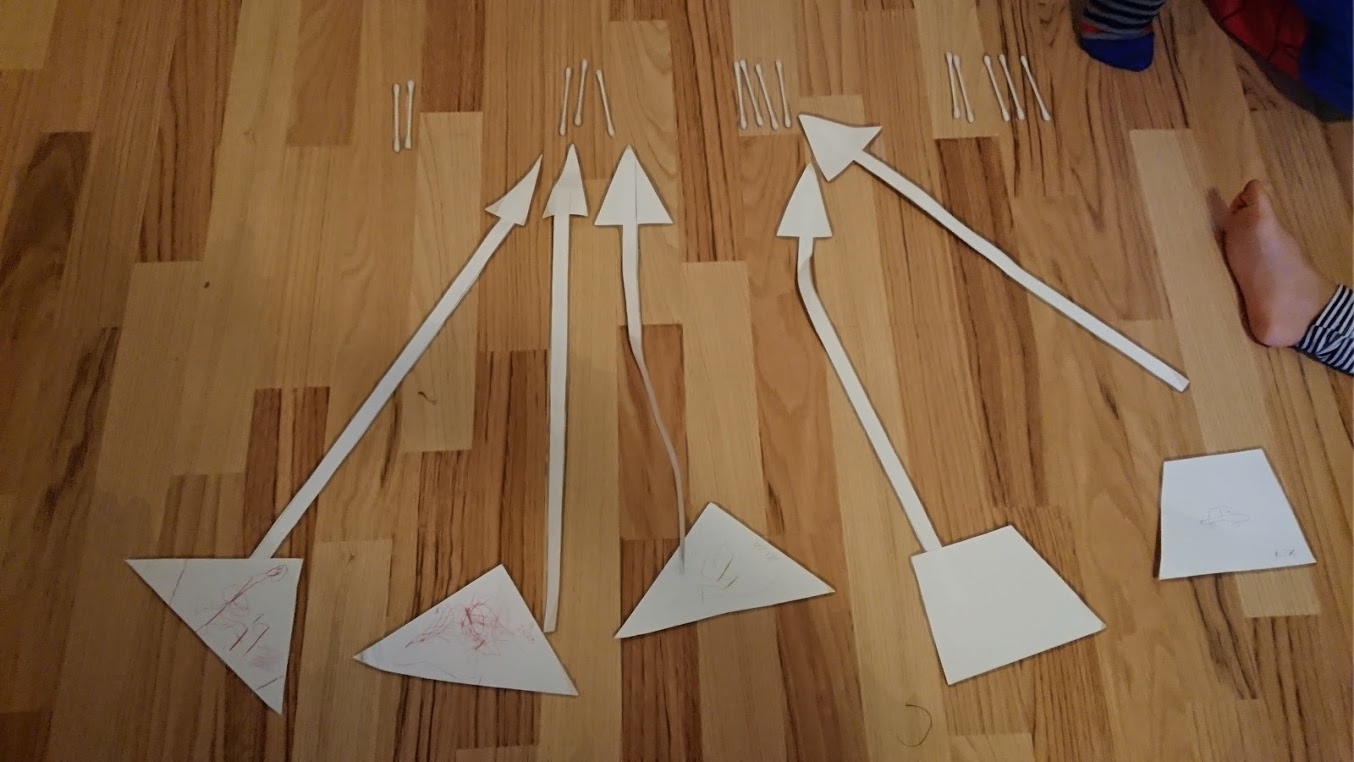
Jana, one of the Epsilon girls, turned four last Saturday so we promoted her from a triangle to a quadrilateral. She cut the following shape out of paper:

We updated the function representing the kids’ ages. Iztok and Jana are now four and so their arrows point from their shapes to the group of four sticks.

Then it was time to play thieves and detectives. I asked who wanted to be a thief. Everybody did. Then I asked who wanted to be a detective. And again, everybody raised their hands. After a while, we managed to agree on who would be thieves and who would be detectives.

The thieves remained in the room and were asked to decide between them who would actually steal an (incredibly precious) lamp.

That person had to dip a finger into magnesium powder and leave his or her fingerprint on a tablet. The tablet was used just because fingerprints are clearly visible on it.

Then the detectives were called into the room.

When the detectives (me included) arrived at the crime scene there were no fingerprints on the tablet. I asked the suspects (the group of thieves) about it, and they said they had wiped them off. Well, yeah. It seemed I hadn’t been clear enough.

So we repeated the procedure. We, the detectives, left the room and returned after a couple of minutes. This time there were some fingerprints!

More precisely, there were two fingerprints on the tablet. We asked each of the suspects to leave a fingerprint on the tablet. We wanted to compare the fingerprints with the two that had been left when the lamp was stolen. That way we should be able to find the thief!

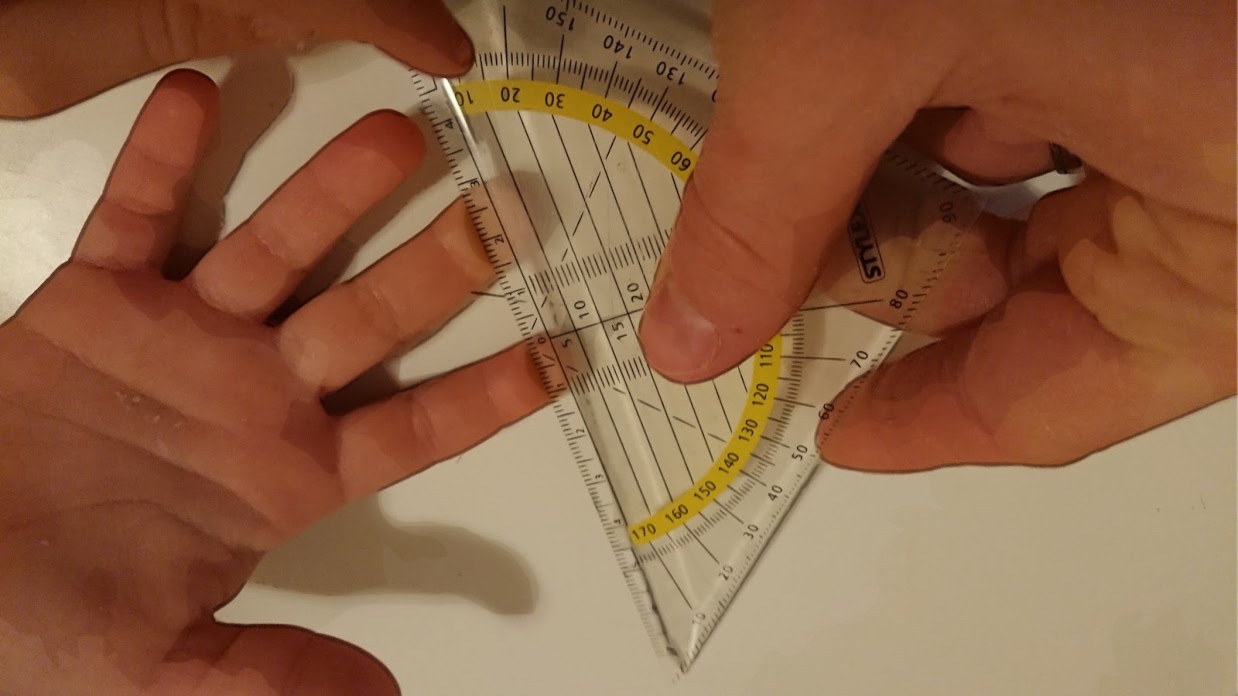
We measured and compared the fingerprints with a ruler. In reality it was hard to say anything as they probably hadn’t used index fingers as they were told, and the fingerprints were actually barely visible (the one on the photo is mine just for demonstration purposes). But they didn’t really care, they just liked playing detectives and thieves.

The last activity was throwing a die. After each throw, we drew a cross in the coordinate system:

At the beginning, I asked which number would appear the most. Vesna said it would be six. When I asked for an explanation, she said: Because it has the most dots. I tried to explain all six options were equally probable, but they started their own discussions, and the coordinate system wasn’t interesting anymore, so I just stopped.

Deltas

Things went smoother in the second group. We first made some measurements with rules – they drew some lines on paper and we measured them. For example, we drew a six-centimeter line representing six years. We discussed how to draw months – one month as one millimeter? We discussed a bit the fact that there are ten millimeters in a centimeter while there are twelve months in a year.

Then we played thieves and detectives. It was funny to see them truly fascinated when I found out who the thief was. There were three suspects, but I actually knew all along who the thief was as two of the suspects had clearly expressed their desire not to be thieves when we started.

We played throwing a die in this group too. Four dots appeared the most times and when asked which die side was going to win, they obviously said four. I said that if we threw it many many times, we would have approximately the same number of crosses for all six sides. Not sure if they believed me though.